As you may have guessed from the previous posts, I’m curious about the processes happening during hard cardio exercises. If you are not interested in the details (or get lost in them) please jump to the conclusion.
The first thing I wondered about is how the heart rate is determined by our body. What makes the heart rate rise? According to the book “Exercise Physiology” by McArdle et al. the heart rate is controlled by our brain stem through the in- and exhibitatory effects of the sympatic and parasympatic nerves. The brain stem in turn receives stimuli from the muscles which tell how hard the muscle is exercising. The brain stem then seems to calculate the required heart rate from that. In the early phase of exercising, the heart rate is dominated by our expectation of exercising. Experienced athletes have been tested and just by thinking of running a sprint they could increase their heart rate to around 75% of the target heart rate.
————————————————————
Variables and definitions:
To test some hypothesis about our heart rate I made a simulation. The simulation uses the following variables:
- Heart rate
- Muscle O2-storage
- Muscle O2-consumption
- Muscle Lactic Acid
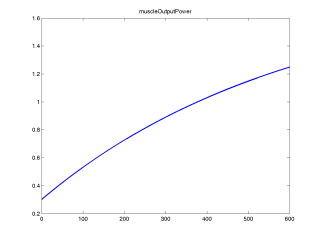
- Power output
- Venous blood O2 volume (CvO2)
and constants:
- Stroke volume = 70 ml
- Arterial blood O2 volume (CaO2) = 20% = 20 ml O2 per 100 ml blood
- Basal O2 consumption (O2 consumption when body is in rest) = 250 ml O2 / min
- Blood volume = 5 L
- Maximum muscle O2 uptake = 850 mL / min / leg (see [1])
These parameters have some limits and lead to derived values:
- Heart rate at rest = 60
- Maximum heart rate = 220
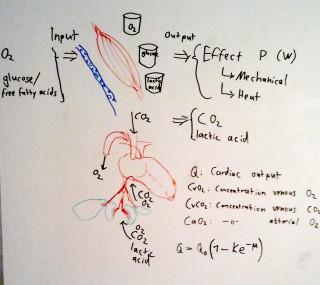
- Cardial output = heart rate * stroke volume
- Cardial O2 output = cardial output * CaO2
- Muscle O2 consumption = constant * power output
- O2 available for exercise = cardial O2 output – basal O2 consumption
We control the power output and watch the effect on the rest of the variables.
Since the heart rate is calculated in a complex way by the brain stem I cannot model the heart rate in an exact manner, but instead I decided to link the heart rate to the O2 volume in the venous blood (CvO2). CvO2 represents how much O2 is consumed and if there is enough of it. Since I use an O2-storage in the muscles in the model CvO2 is calculated in two different ways, depending on if there is O2 in the storage of the muscle or not.
If the O2 in the muscle’s storage is sufficient to supply the muscles O2 consumption, then the storage is only refilled:
If the storage is not sufficiently filled, O2 from the blood has to contribute to the O2 supply of the muscle and if there is not enough O2 in the blood either, the muscle acid will increase.
- The body tries to sustain an aerobic environment, i.e. it tries to keep the muscle O2 storage filled.
——————————————————————————–
Results:
I increased the muscle power output in the following scheme (1 is the maximum power output at which the muscle can uptake enough O2 to stay aerobic).
The simulation outputs the following plots for some of the variables
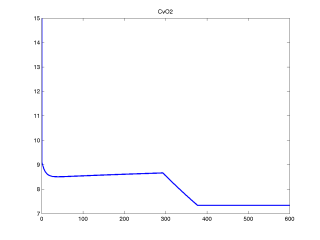 |
| CvO2 |
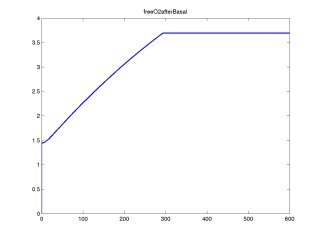 |
| O2 available for exercise |
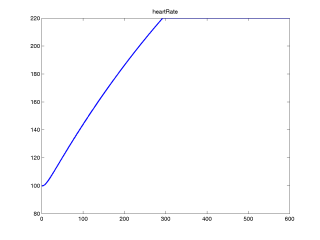 |
| Heart rate |
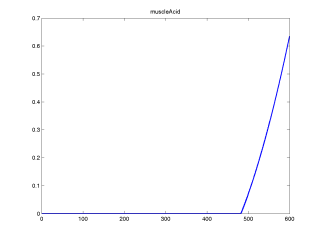 |
| Muscle lactic acid content |
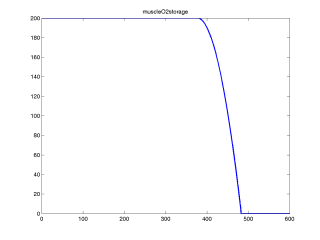 |
| Muscle O2 storage level |
—————————————————
Conclusion:
From the plots above we note that the heart rate hits maximum before the muscles reach their O2 uptake limit, which clearly is not the case when exercising. Also the lactic acid only starts to build up long after the heart rate hits maximum, which of course is wrong as well.
The reason why the heart rate in this simulation hits it’s maximum so early is because we assumed that the body tries to sustain an aerobic environment, where there is sufficient O2 for the muscles. An increased heart rate supplies the body with more oxygen.
What I conclude from this simulation is, that the body does not try to sustain an aerobic environment by all means. Instead the body seems to find a balance to hold down the heart rate and accept more lactic acid to build up and let the muscles O2 storage empty more. Also this simulation does not take into account the effect of the lactic acid build-up, which would result in a decreased efficiency of the muscles.
Matlab-script: Download Matlab script
[1] Blomstrand, Rådegran and Saltin, “Maximum rate of oxygen uptake by human skeletal muscle in relation to maximal activities of enzymes in the Krebs cycle”, Journal of Physiology (1997), 501.2, p. 455-460
[2] http://www.ptdirect.com/training-design/anatomy-and-physiology/adaptations-to-exercise/acute-cardio-heart-responses-to-exercise
Comments are closed.